

Given triangle ABC, and P being any point on the plane, the pedal triangle is formed by constructing the perpendiculars from P to the sides of the triangle. The three intersection points, R, S, and T, are the vertices of the pedal triangle. A GSP script tool can be found here.

We can then create the circumcircle for triangle ABC. If we place the pedal point on the circumcircle, the pedal triangle will degenerate into a single line. This line is known as the Simson Line (shown in blue). The original triangle is given in red lines. The perpendiculars are shown here in yellow. The circumcircle is in dark green.

We can also find the midpoints of each side of the pedal triangle. If this is done prior to creating the Simson Line, we can animate the pedal point to follow the circumcircle. If the midpoints are traced, the light green ellipses are formed. The GSP file can be viewed here.

The pedal triangle of the pedal triangle of the pedal triangle of a point is similar to the original triangle. In other words, A’B’C’ is the pedal triangle of RST of the pedal triangle XYZ of the pedal point P is similar to ABC. So triangle A'B'C' is similar to ABC.


Using the script tool given above, you can easily make this construction in Geometry Sketch Pad (GSP), so we know that we can indeed create this construction. We can then construct the circumcircle of each triangle using the following script tool.
An important property of the circumcircle that is vital to this proof is that given triangle ABC with the lines tangent to points B and C on the circumcircle is shown below. It will be proved below this proof using Euclid’s Propositions III.20 and III.21 in addition to an extra case that will be proven.
So then we can construct the circumcircles around triangle BYZ, triangle YTR, triangle TXS, triangle B’RC, and triangle A’CS. To use the property of the circumcircle described above, we need to construct the segments PB, PY, PT, PX, PB’, PA’, PC’, PR, and PS. Then using the property of the circumcircle described above and the segments we created, we can see that angle YBP = angle YXP = angle TXP = angle TSP = angle A’SP = angle A’C’P. Also we can see that angle PBX = angle PYX = angle PYT = angle PRT = angle PRB’ = angle PC’B’.
Since angle PBX = angle PC’B’ and angle PBY = angle PC’A’, and since angle ABC = angle ABP + angle CBP and angle B’C’A’ = angle B’C’P + angle A’C’P, we can say angle ABC = angle B’C’A’ for they are made up of each parts.
Similarly we can repeat this process for each angle of triangle ABC to get angle ABC = angle A’C’B’ (found above), angle BAC = angle C’A’B’, and angle ACB = angle A’B’C’.
Hence since the angles of triangle ABC and A’B’C’ are equal, the triangles themselves are similar.



Proposition III. 20: In a circle the angle at the center is double of the angle at the circumference, when the angles have the same circumference as a base.
Proposition III.21: In a circle the angles in the same segment are equal to one another.
Making the pedal points the same as various centers of triangle ABC
If the pedal point is the centriod of the triangle ABC:

If the pedal point is the incenter of the triangle ABC:
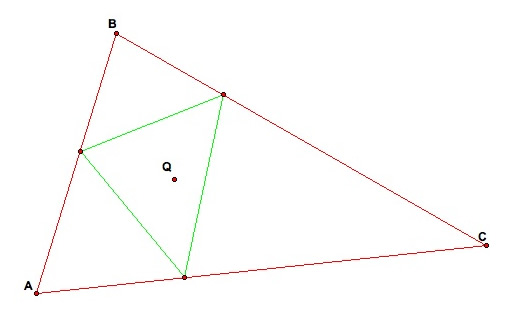
If the pedal point is the circumcenter of the triangle ABC, then the medial triangle is created in green:

If the pedal point is the orthocenter of the triangle ABC, then the orthic triangle is created in green:
